Stodola-Vianello Method for the Buckling Load Analysis of Euler-Bernoulli Beam on Pasternak Foundation

Abstract

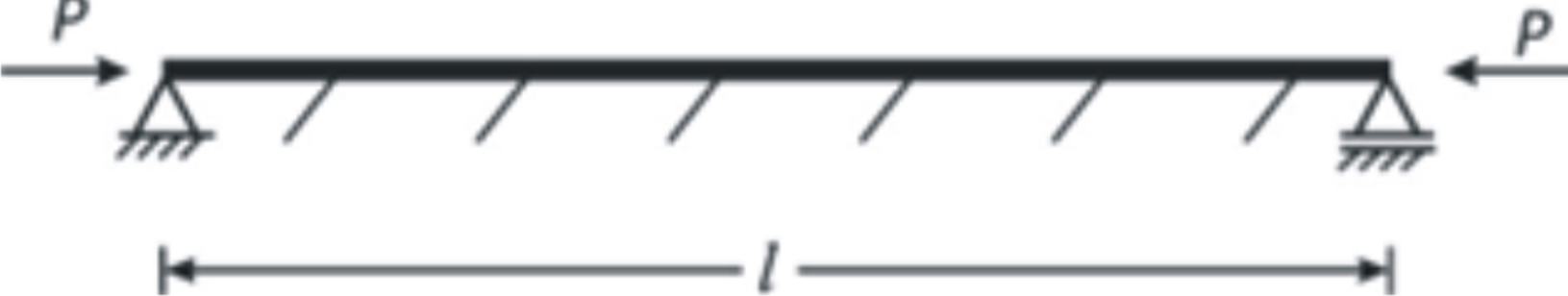
The determination of critical buckling loads of thin beam on Pasternak foundations (BoPF) subjected to in-plane compressive loads is vital to their analysis and design. This study presents the Stodola-Vianello iterative method for formulating and solving the governing ordinary differential equation (ODE) subject to the boundary conditions. The governing boundary value problem is expressed in iterative form using the method of four successive integrations after re-arranging the ODE. A suitable buckling mode is employed in the derived Stodola-Vianello iteration formula for the pinned-pinned end conditions studied. The convergence requirement of the nth buckling mode is used to derive the characteristic buckling equation whose roots are used to obtain the buckling loads at the nth buckling mode. The obtained expression for the nth buckling mode was found to be exact because exact buckling eigenfunction was used in the derivation. The critical buckling load was found to be exact and correspond to first buckling mode. The critical buckling load expression was expressed in standard form in terms of critical buckling load coefficients which was found to depend upon the beam parameter and parameters of the Pasternak foundation. The values of the critical buckling load coefficients were found to be in close agreement with previous studies. Exact buckling load solutions were obtained for all the buckling modes of the BoPF, and the critical buckling load was found to be identical with exact critical buckling load solutions obtained by previous researchers.